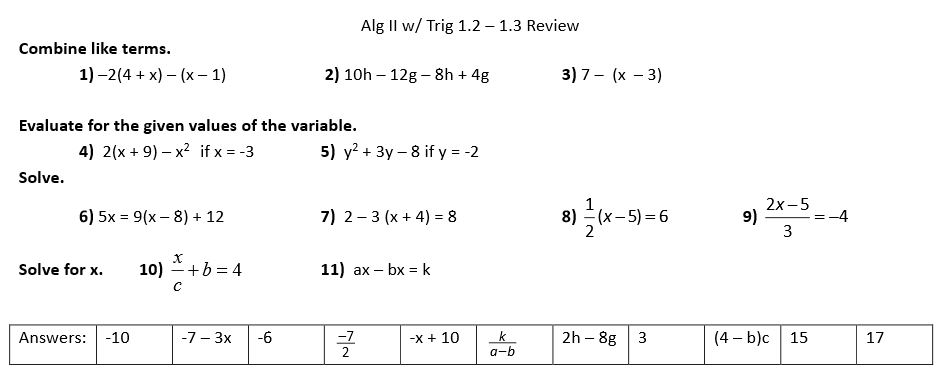
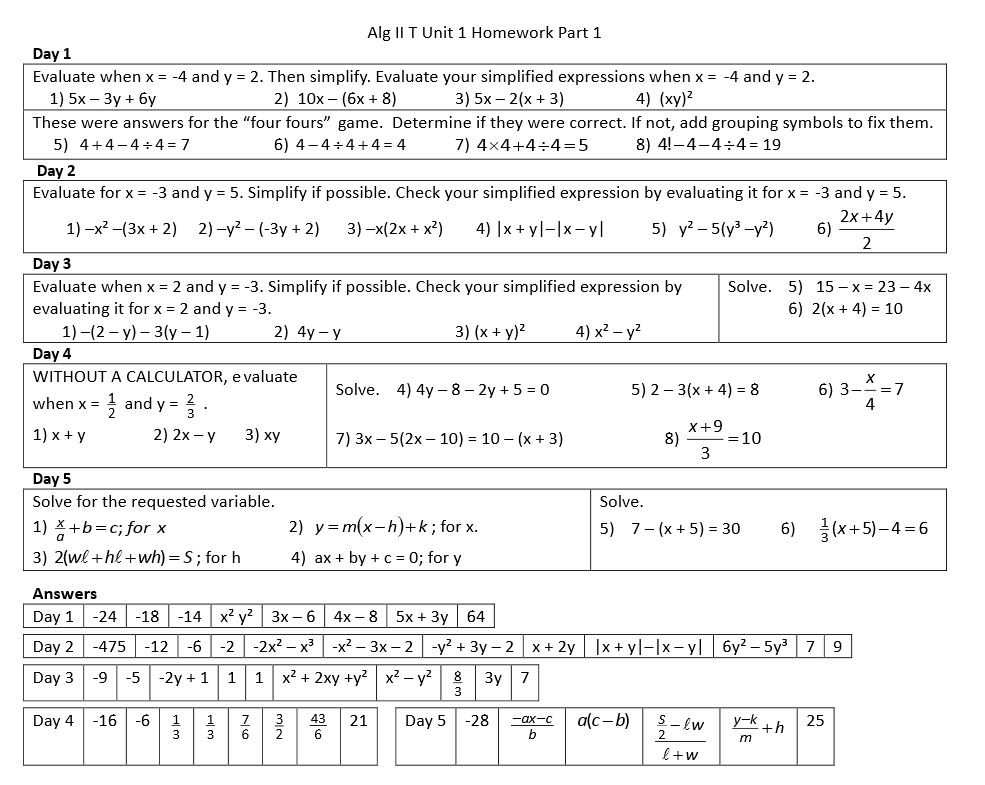
Last weekend, there was some twitter chatter about making life-size graphs so students could explore points/transformations/what-have-you. That got my wheels turning, and after a quick trip to the dollar store and about an hour’s worth of work I ended up with:
Steps:
1) Buy four shower curtains at the dollar store. Bonus if they are prelined!
2) Tape together with packing tape. (hint: tape them down to the floor with washi tape to hold them down, then tape the seams on the front. When done with the whole thing, flip over and tape the back)
3) Use duct tape to mark the x-axis. Print labels (file here), cut out, measure your axis, and tape down with packing tape.
4) Apply colored masking tape for 1/2, root 2/2, root 2/3. (see next picture since the masking tape was at school)
Total cost depends on how much tape you have around the house. I used almost 3 full rolls of colored masking tape, but the good news is I bought it from naeir.org. Have you heard of this site? One of the teachers at school shared it with me. Basically companies donate overstock and you get to buy it for the cost of handling. You do have to spend at least $25 and shipping takes about 2-3 weeks, but holy cow, can you get a lot of stuff for $25! My first shipment I got 8 rolls of patterned/colored masking tape, 2 packs of 12 small post-its pads, 8 post-it pop-up cubes, 12 correction tape thingies, a pack of sharpies, 3 sets of dividers, 2 packs of post-it labels, and I think some other things I’m forgetting. It’s crazy!
Anyway, in class, I handed out dry-erase pockets with a sheet that had an x value in it (0, pi/6, pi/4….2pi) (file here) and told the students to find sin x, 2 sin x, sin 2x, sin1/2x, and cos x. Holy moly. We could have easily spent the day doing that. No, if x = pi/3, sin2x does not equal 2pi/3. Once we got that sorted, we went out into the hall. I had all the students stand on their x-coordinate, then step to the y for the function I called out. It was very easy to find people who made wrong calculations! 🙂 Here’s what cosine looked like:
And sin 1/2x (with an outlier!)
By third time I ran through it, I had worked some of the kinks out:
- In my first class, I had more people than x-coordinates, so I gave coordinates that were more than 2pi. This did not go well. They were way far down and we couldn’t really see the pattern continuing. The next class I handed out 2-3 points per group and had them work together to find the values, then as we graphed we substituted people in who hadn’t graphed yet. (The class shown had just one person extra.)
- I only did each graph once. We talked about max/mins, who didn’t move and why, how many cycles fit on the mat, etc. I think it would have been beneficial to do sin, then cos, then switch back and forth faster and faster. Then do the same for 2 sin x and sin x, sin 2x and sin 1/2x, etc. And also positive and negative. The last class we even tried sin x + 2 (“oh, that means we need to all step up 2!”)
- Have them write down noticing/wonderings as we are doing it, or a quick sketch of the graph (maybe have some axes printed on the back of their point card?) to help solidify the concepts.
The next day, when we went to graph, I asked them if it helped to visualize what we did yesterday. Only a few raised their hands. I told this to Mr Craig, wondering if I would do it again or if it would be more efficient to just jump into graphing then practice. He said, “Hey, you helped those 5 kids see it better! Plus sometimes it’s about the experience, not about being efficient.” Sometimes that Mr Craig can be pretty smart. (Don’t tell him I said that, his head is big enough already.)
In other news, this happened on Twitter the other night:
Do you think I will hit 1,000 followers or 10,000 tweets first?
